Measuring Time

     Our family has a large cloth Advent calendar with pockets sewn on each day. The kids always took great delight in moving a little felt mouse from pocket to pocket leading up to Christmas Eve. It built suspense but also served as a way of keeping track of 'where in time' we were relative to the big day. Helping our children develop a sense of time and natural rhythm is a great gift. It's comforting to know what to expect and when to expect it. Patterns are natural, both as a physical reality-- sunrise, sunset; fall, winter, spring, summer; weekly rhythms, etc-- and as a psychological reality too. We all need to be able to find our way in time as well as space.

     The calendar pictured here was made by five year old Zach who took great pleasure in choosing pictures, filling in the month name, and numbering the days. This was a monthly project, but a daily exercise in noticing the number of the day, the day of the week, and how many days to go to some given event-- Christmas, birthday, Saturday soccer game, and so on.

     There are dozens of books for children that address the business of time and seasons. Here are some examples:
Pajama Time (Sandra Boynton)
The Best Time of Day (Eileen Spinelli)
What Time Is It, Mr. Crocodile? (Judy Sierra)
The Year at Maple Hill Farm (Alice and Martin Provensen)

     Developing a 'sense of time' on a smaller scale is also important and can be done with all sorts of games. A simple stopwatch makes a wonderful toy and a great tool for experimenting with time. Events can be timed. A game of prediction involves starting the stopwatch behind your back then trying to stop it at 30 seconds or at least seeing how close you get to the target time. Similar games can be played with timer clocks. Sand dials, digital watches and clocks, traditional toy clocks, etc, are all helpful. Learning about time should be natural and gradual and 'part of the landscape' so to speak. The dog has to be walked at eight. It takes an hour to drive to Grandma's house. The cookies go in the oven for fifteen minutes. It's easy to take all that for granted and forget that it needs to be verbalized for our children.

In the Bag

     There is a useful game described in Patricia Davidson's Idea Book for Cuisenaire Rods at the Primary Level. A child, with his hands behind his back, is given a rod and asked to guess the color. This gives the little person a very tactile sense of the relative sizes of the rods. We found that identifying the very large and very small rods was not so difficult, but distinguishing dark green from black was much harder. The game was fun and helpful in developing real familiarity with the rods.
     As a variation on this theme, we made a game of placing a bunch of rods of all sizes in an opaque cloth bag. The first version of our game required the player to grab a rod (unseen) and predict the color before she removed it from the bag. When this became too 'easy', the mission became finding a specific color rod in the bag and pulling it out. Another variation had the player building a staircase and then a reverse staircase-- in other words, rods of ten different sizes had to be pulled out of the bag in order from smallest to largest then from largest to smallest. This is easier if there are only ten rods in the bag (ok as a beginning version) but harder when there are three or four or five of each color in the bag.

     These sort of tactile games are more important for some children than for others, but I have found them useful all around. Children with learning disabilities or deprivations of various sorts seem to especially benefit from the exercise. I once worked with children so culturally deprived they couldn't, at ages five and seven, recognize themselves in a photograph or understand two dimensional representations so as to make sense of a story book. They were enormously helped by a French game called Tactilo which I can't seem to find anywhere now. It was by Fernand Nathan and consisted of beautiful little wooden shapes-- cube, sphere, egg, cone, mallet, bowl, mushroom,etc and cards with matching two dimensional pictures. The object was to reach into a little silk bag and pull out objects to match the flat pictures. It's easy to take skills for granted that little ones need to learn in one way or another in order to move forward in math. And it's also why it's important to allow for plenty of block play, math games, spatial relationship and pattern learning to take place before "jumping the gun" into formal numerical instruction.
     When I was, recently, discussing the 'bag' game for Cuisenaire rods with my daughter, she made the suggestion that the game could be adapted to Pattern Blocks as well for the purpose of learning the shapes by feel. Sounds like a great idea to me.

What Fits?

     This is a Cuisenaire Rods game that lets your child begin to 'see' or 'feel' or 'sense' math relationships. The object is to start with a given rod, say the orange one, and 'fit' other rods to it.  Two yellows fit. A dark green and a purple fit, too. Ten white rods fit. And so on.

     No numbers need to be assigned, but they will come eventually. An orange rod is ten units long and the rods that 'fit' are the numbers that add up to ten. I think it's important to just go with colors and size and feel for quite a long time and let the game be open-ended. When arithmetic finally does come up it will seem perfectly reasonable that 5 + 5 = 10 because "of course" yellow and yellow fit orange. I find it helps some children to verbalize the relationships just that way: "Look, Mom-- brown and red make orange"  or "Green and red fit yellow."

     Cuisenaire sells a little frame with their basic sets that make 'fitting' the rods easier for little people. This frame is especially useful if the game is amplified to include more than two pieces in a 'fit' and if the 'fits' are stacked up into a pattern or design. These 'rugs' are a lot of fun to create. Some pictures of individual fits as well as rugs follow.

     As children get older and more experienced, the game can be amplified to include 'fits' to double length rods. For instance, the question could be asked, 'What fits two brown rods?' Fits can also be more challenging if the player is limited to a single color answer. For orange this would require two yellow rods or five red rods. For 'odd' size rods, like yellow, there is no single color answer. That problem is solved if a single white rod is permitted when needed. In that case, yellow could be fitted with two red rods and a white.

Mathland Weddings

     This game introduces the ideas of even and odd. The photos here use white Cuisenaire rods, but literally any kind of manipulative will work as well. Note that the game does not require that the child already understand numbers in the formal sense.
     Given a handful of blocks, demonstrate pairing until all the pieces are used up. If everything pairs, the group is ‘even’. If one is left over, the group is ‘odd’. Five year old Rebekah suggested that the ‘extra’ block was the ‘priest’ performing the wedding ceremony. So, if there’s a ‘priest’, the group is odd. No ‘priest’ means the group is even.                                       
This group is even. There is no left-over block. The second group is odd. There is a 'priest' to perform the ceremony. The third group is also odd.
 


     For children who can count to five and can understand the notion that ’five’ is the number of these blocks, you can introduce the idea that ‘five’ is always an odd number. Your little person can experiment with a variety of objects to verify that five toy cars, after the pairing process, will still leave one car left over. The same process can be used to examine ‘four’ which is always even and ‘three’ or any other number. The number ‘one’ might be a challenge but makes for interesting discussion. In Rebekah’s case, the reasoning went something like this: “Nobody married nobody but there was still a priest in the church so it was odd.” We didn’t bother with ‘zero’ at that time, but if we had, the discussion would probably have come to a conclusion something like this: “It’s always odd if there’s a priest and if there’s no priest it’s even. So if nobody marries nobody and there’s no priest either, then its even.”

This is a more challenging group because of it's size.
     There are many other possible variations on this game. The priest might be a rabbi or preacher instead. Or, since little boys don’t always get into the ‘wedding’ thing, the whole game can be turned into making pairs of shoes or boots for imaginary people, soldiers, whatever. When the blocks all pair, the group is even. A left-over boot means the group is odd.

The Inside of the Inside: a Cut and Paste Project

     This is an activity for children who have enough dexterity to use scissors and enjoy craft projects. The objective is to take a page or part of a page from an old magazine and cut out a piece then cut out a second piece from the new piece. (Pictures follow.) The child can then create a collage with the pieces. The project can be either abstract– just a colored piece of paper or a page with an abstract design, or it can be fairly concrete– an object within an object.
     The point of the exercise may not be obvious, but it helps a young person learn about wholes and parts, relative locations, and that the pieces are smaller than the original whole– ‘the inside is smaller than the outside’ idea. It’s a project that blends math and art and also lends itself to further elaboration for little people that enjoy the activity. For instance, the number of insides can increase or the cut-out shapes can take on more complexity.
 
Step 1: Collect a piece of a magazine page, a sheet of plain paper, scissors and glue.

Step 2: Cut a piece from the magazine page. The page can be creased to make it easier to start the cutting process.














Step 3: Cut a smaller piece from the first cut-out.












Last Step: Paste the pieces on the paper sheet.

Here are a couple of examples of more 'concrete' designs: 
 
 
 
 
 
 
 
 
 
 
 
  
 
 
 
 


 
A note on the conservation of matter: Your little person can re-assemble the pieces before the glue-down step to begin to understand that cutting and taking out pieces doesn’t result in ‘more’ or ‘bigger’ except in the sense of ’spread apart’. This idea is much less obvious than you might think.
     More pieces, more complex design:  


 
 
 
 
 
 
 

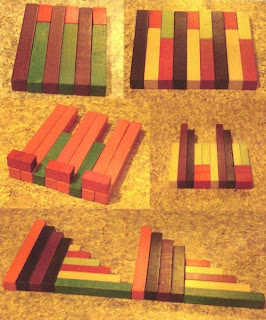
Pattern Trains

     The object of this game is to copy or continue a ‘train’ pattern. I like using Cuisenaire rods for this game because of their versatility and potential for gradual increase in complexity– but the basic idea works with any number of materials including pattern blocks, building blocks, Legos, buttons, bottle caps, coins, and so on.
     The pictures that follow are pretty much self-explanatory, but I do have a few general suggestions. First of all, keep it fun– don’t frustrate. Reverse roles with your little person sometimes and let him create a ‘train’ for you to continue. Obviously, don’t use coins or very small objects with very young children who might swallow them. (Note- coins can be washed and probably should be.) And finally, I have found that verbalizations are very helpful to understanding a sequence. For example, a very simple train might be a sequence of alternating red and white blocks. Saying or chanting “red-white, red-white, red-white” as you lay down the pattern helps clarify the intent. Your little person can continue the “red-white, red-white, red-white” chant as she continues the train.
     As train sequences become more complex, not only color and size can be varied, but positioning variations (vertical, horizontal) can also be used.

Red-Yellow, Red-Yellow, Red-Yellow...

White-White-Red, White-White-Red...


 

Purple Up-Red Down-White, Purple Up-Red Down-White, Purple Up-Red Down-White...


 

Green Up-White-Green Down, Green Up-White-Green Down, Green Up-White-Green Down...


 

Yellow-DoubleRed, Yellow-DoubleRed, Yellow-DoubleRed...


 
 
White-White-White-DownPurple-UpRed, White-White-White-DownPurple-UpRed...

 
   

 
 
 
 
 
 
 
 
 
 

Pattern Block Trains


 
 
 
 
 
 
 
 
 
 
 
 
 
 

 

More Pattern Block Trains


 
 
 
 
 
 

Lego Trains (More Views of the Same Trains Below)


 
 
 
 

 
 
 

 
 
 
 
 
 
 
 
 

 
 
 
 
 
 
 
 
 
 

 
 
 
 
 
 
 
 
 
 
 
 

Coin Trains: Dime-Penny-Penny, Dime-Penny-Penny..., Penny-Quarter, Penny-Quartr..., and Penny-Penny-Penny-Penny-Penny-Nickel, Penny-Penny-Penny-Penny-Penny-Nickel... or, One-Penny-Two-Pennies-Three-Pennies-Four-Pennies-Five-Pennies-Nickel, One-Penny-Two-Pennies-Three-Pennies-Four-Pennies-Five-Pennies-Nickel...

Hungry Joe

     I invented Hungry Joe years ago as a way to work with very young kids on math comparison ideas. It simultaneously introduces the math symbols to go with the concepts.

     All you need are three index cards with a hand-drawn face on each:

     These are used to play a game. Hungry Joe has a crazy appetite. He always tries to eat the larger object or quantity and his ‘mouth’ opens accordingly. When two objects or quantities are equal Hungry Joe is at a total loss and his mouth just drops open into an equal sign. So if I place a large apple and a small one on the table, the object of the game is to place the correct Hungry Joe face between the apples. A large apple on the child’s left and a small on the right requires the Joe card in which the ‘open’ end of his mouth is facing left.
     The same game can be played with counters, cuisenaire rods, toys of various sorts, glasses of water or juice, M&M’s, building blocks, real world objects– big chair vs little chair or two chairs vs five chairs– and so on. Some examples (exploring both size and number) follow:

Hungry Joe will choose the two-chair option for his meal.





Oops! Hungry Joe can't decide because both options are the same.

Hungry Joe will definitely go for the two cans.







Four forks beat three.

 

The big vehicle makes a better meal than the smaller.   

Those fishies are the same size!
 

 A big turtle beats a little one. 



     More sophisticated objects/ideas can also be introduced when appropriate, for instance a dollar bill on one side, three quarters on the other and eventually regular numerals, fractions, or decimals can be used.
     Remember this should be fun or it won’t really be helpful. Your little person may want to draw his/her own Hungry Joe faces or pose problems for you to solve. Taking turns works well.
     Eventually a revised ‘Hungry Joe’ in which only the mouth on the face remains can be used as a transition to using the ‘mouth’ images as stand-alone symbols. Here are a couple of examples:

Have fun– make up your own variations.